dedicated to explicating prime numbers
without imaginary numbers

Polynomial Pronic Alignment

Seen in the Sacks Number Spiral, all polynomials of type N^2 have a fascinating property in common that the traditional parabolic graphing of polynomials (in the Cartesian plane) does not show - namely, pronic alignment on the third coefficient.

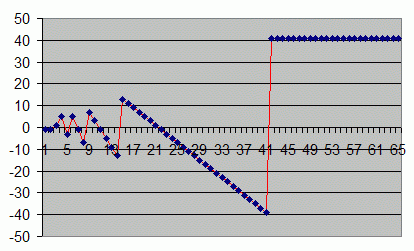
Alignment with the pronic axis of the spiral occurs when the number of terms exceeds the third coefficient. So, for example, in the case of n^2+n+41, the alignment occurs on the 42nd term (value 41), when the result of the expression is 1763. This is the first result to have an offset of +41, with the preceding offset being -41, both from the pronic number of 1722. It should also be noted that 1763 is a composite with the prime factors of 41 and 43 - the first two results of the polynomial expression!

The pronic offset number of the polynomial is simply determined by the sign and number of the third coefficient of the polynomial. A negative coefficient produces a negative offset. To better understand how this works, try any N^2 polynomial in Vortex. Or to see how it works in QTest, press the O key when you click Generate. Before this offset stabilizes, the polynomial moves from positive to negative offset and back again. This appears chaotic, but when viewed in the perfect square-aligned (and hence, pronic-aligned) spiral, this is in fact an orderly rotation that eventually flattens out, paralleling the pronic axis. Larger constants mean larger offsets that mean more rotations.

It appears that without exception the greatest concentration of primes for any polynomial - including the uninterrupted prime sequence of a perfect prime polynomial like n^2+n+41 - occurs before it aligns with the pronic axis.